Rhythm Lectures

#2 - Subdivisions and Tuplets

Overview

The purpose of this episode is to introduce the fundamental concepts required for further study. These concepts will be used for all subsequent episodes. They are:

The basics of subdivisions
Subdivisions as grids
An introduction to tuplets

Where the beat divides a span of time into equal segments, subdivisions divide the space of time between

the beats themselves. Subdivisions describe the division of the beat into evenly sized segments, denoted by a number. A two-note subdivision divides the beat into two (even) parts, while a six-note subdivision divides it into six.

I. Subdividing the Beat

In the previous episode, we discussed the concept of the beat, specifically in a metric sense. This beat essentially serves to divide time into regular chunks, the frequency of which is denoted by the tempo. Subdivision, therefore, divides the beats themselves.

The subdivisions describes the number of parts into which the beat has been divided, and it is incumbent within this that the division is into equal parts; this is one of the fundamental attributes of subdivisions. A two-part subdivision divides the beat in half; a three-part into thirds, and so on. As the tempo remains consistent, it is therefore the case that higher subdivisions create a faster rhythm relative to the beat; higher subdivisions require more notes to be played within the same time frame, just as dividing a circle into more pieces demands each piece to be smaller.

When the tempo is the same, then, a 2-part subdivision will be perceived as slower than a five-part subdivision, and vice versa.

If we take quarter notes as our starting point, the notational structure allows for common subdivisions through the common note values. 8th notes represent a two-part subdivision; 16th notes a four-part, and 32nd notes an eight-part, and so on. Rhythm is created by the combination of different subdivisions within the measure or passage of music.

There are a few points to note here. Firstly, rests posses the same value is notes, such that we can conceive of a two-part subdivision where one of the two parts is an 8th note rest. In this way, we can play three notes within a beat in what is actually a four-part subdivision. The easiest way to conceptualise this is with a 16th note subdivision, the fourth of which is 16th note rest. The subdivision is 16th notes, but only three of them are actually played.

Secondly, there is no inherent link between subdivision and note value. The previous examples assume the quarter note as the beat, linking two-note subdivisions to 8th notes, and so forth. This need not be the case. In a bar of 7/8, a two-note subdivision would refer to 16th notes, while in 3/2, quarter notes would represent a two-part subdivision. While the rhythms may be perceptibly identical, the note values used are linked to the time signature, and not the subdivision itself.

This brings us onto the concept of grids.

II. Plotting Rhythm

I have already stated that rhythms are created by combining subdivisions against the same underlying beat. For instance, a simple rhythm can be created by alternating between a two- and a four-note subdivision. Notwithstanding the previous point about the link between subdivision and note value, in 4/4 time, this rhythm would be written using 8th notes and 16th notes.

Using the concept of subdivision, we can establish a grid upon which any rhythm is based. This grid will correspond to the smallest note value within the rhythm. Taking the clave as an example, written in one bar of 4/4, the clave contains the 16th note as its lowest value; this tells us that a 16th note grid is required on which to plot the rhythm.

Taking this as our example, we begin with a bar of 4/4. This tells us that there are four quarter notes per bar, and, as a binary time signature, these quarter notes represent the beat itself. We can then subdivide the beat into 16th notes, but rather than playing all of these 16th notes, we can conceive of them as possible locations into which a note can be placed. As the clave is based on a 16th note grid, every note of the clave will fall into one of these slots.

To see this, we can create the clave rhythm while counting 16th notes, and in so doing, we see that every note of the clave aligns with one count of the 16th notes. In other words, every note of the clave is, conceivably, a 16th note. However, to avoid writing out an inordinate number of 16th note rests, we use longer note values where possible for the sake of legibility.

In this context, the 16th note grid is the only one that will allow for the clave. If we remained in 4/4 but tried to use a different subdivision, say quintuplets (a five-note subdivision), we would not be able to plot the notes of the clave accurately. The clave itself is a predefined rhythm. This means that the space between the notes is fixed, and changing the spacing changes the rhythm. In one bar of 4/4, it is not possible to plot the notes of a clave on a quintuple grid and maintain the correct spacing between the notes.

Musicologists sometimes plot rhythms on circles, with the underlying pulse of the rhythm represented by evenly space points around the circle, like a clock face. In this fashion, the clave is plotted on a circle with 16 points, five of which are occupied by the notes of the clave itself. This format is completely independent of note value or time signature, simply dividing the rhythm in such way as to accurately show the spacing between notes. It is, of course, no coincidence therefore that we plot a clave on a 16th note grid in one bar of 4/4.

The time signature of a piece has some bearing on the subdivisions used within a piece of music. A ternary time signature like 6/8 would imply the common use of three- and six-note subdivisions, corresponding to 8th notes and 16th note respectively, but again, this link between subdivision and note value is solely contextual. This also gives some hint as to why one time signature may be chosen over any other. When we take a common tune like humpty dumpty, we can hear very quickly that it is based on a three-note subdivision. It therefore makes much more sense to write this in a ternary time signature, than to write it in a binary time signature with tuplets.

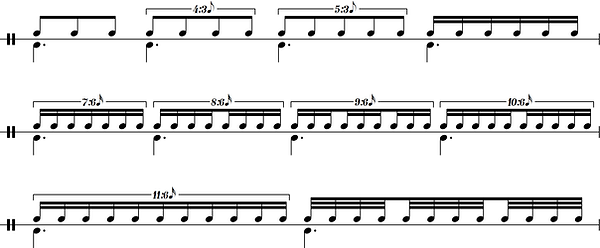
III. Tuplets

Within a metric context - within a time signature - there are native and non-native subdivisions, dictated by the note value used to represent the beat. In a 4/4 time signature, quarter notes are used to represent the beat, and the nature of quarter notes give rise to native subdivisions. These native subdivisions arise from the dividing in half of the quarter note.

Quarter notes, by definition, divide in half to create two 8th notes. These, therefore, represent a two-note subdivision. Quarter notes likewise divide into four 16th notes (giving a four-note subdivision), and eight 32nd notes (giving an eight-note subdivision). As these subdivisions are all derived from the natural divisions of the quarter note, they can be said to be native.

In a ternary time signature like 12/8, the process is the same, except beginning with a dotted quarter note. As this, by definition, contains three 8th notes, six 16th notes, and twelve 32nd notes, all derived from the natural divisions of the dotted quarter note, three-, six-, and twelve-note subdivisions can all be said to be native.

To achieve a subdivision not native to the time signature requires a tuplet. These are modifications with the format x in the space of y, with x and y given numerical values. The former refers to the desired subdivision, and the latter to the most recent native subdivision. A tuplet of 3:2, therefore, reads 3 in the space of 2, where the reference is the most recent native subdivision. An 8th note triplet in 4/4, therefore, reads 3 8th notes (the desired subdivision) in the space of 2 8th notes (the most recent native subdivision.

8th note triplets:

Binary subdivisions:

Subdivisions in 7/8:

Ternary subdivisions: